Article: Geometric approach to optimal nonequilibrium control: Minimizing dissipation in nanomagnetic spin systems

Grant M. Rotskoff, Gavin E. Crooks, Eric Vanden-Eijnden Phys. Rev. E 95 012148 (2017)

[ Full text | Journal | arXiv ]

Abstract

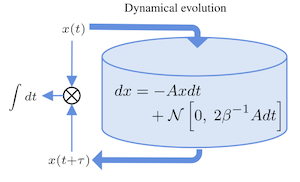
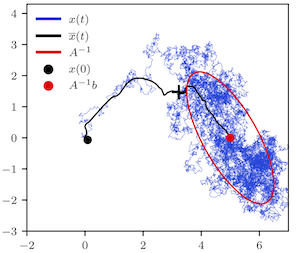
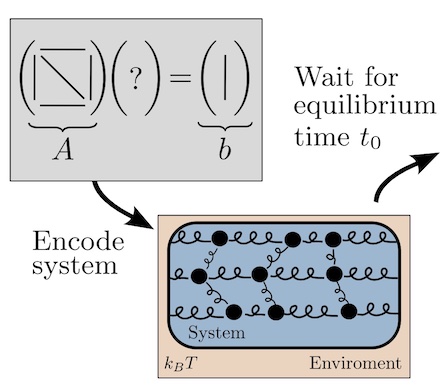
Optimal control of nanomagnets has become an urgent problem for the field of spintronics as technological tools approach thermodynamically determined limits of efficiency. In complex, fluctuating systems, like nanomagnetic bits, finding optimal protocols is challenging, requiring detailed information about the dynamical fluctuations of the controlled system. We provide a new, physically transparent derivation of a metric tensor for which the length of a protocol is proportional to its dissipation. This perspective simplifies nonequilibrium optimization problems by recasting them in a geometric language. We then describe a numerical method, an instance of geometric minimum action methods, that enables computation of geodesics even when the number of control parameters is large. We apply these methods to two models of nanomagnetic bits: a simple Landau-Lifshitz-Gilbert description of a single magnetic spin controlled by two orthogonal magnetic fields and a two dimensional Ising model in which the field is spatially controlled. These calculations reveal nontrivial protocols for bit erasure and reversal, providing important, experimentally testable predictions for ultra-low power computing.